Solving one-step equations is a fundamental concept in algebra, crucial for building a strong foundation in mathematics. These equations involve a single operation, such as addition, subtraction, multiplication, or division, and can be solved using basic algebraic manipulations. Understanding how to solve one-step equations is essential for advancing to more complex mathematical problems. In this article, we will delve into the world of one-step equations, exploring their definition, types, and methods for solving them, as well as providing practical examples and applications.
Key Points
- Definition and importance of one-step equations in algebra
- Types of one-step equations: addition, subtraction, multiplication, and division
- Methods for solving one-step equations, including inverse operations
- Practical applications of one-step equations in real-world scenarios
- Common challenges and tips for solving one-step equations
Understanding One-Step Equations

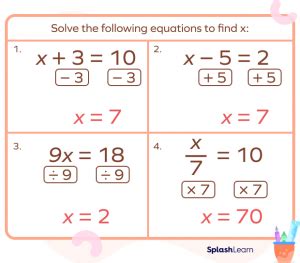
A one-step equation is an equation that can be solved in a single step or operation. These equations typically involve a variable (such as x) and constants on both sides of the equation, with the goal of isolating the variable. One-step equations can be categorized based on the operation involved: addition, subtraction, multiplication, or division. For instance, an equation like 2x = 6 is a one-step equation because it can be solved by performing a single operation, in this case, division by 2.
Solving Addition and Subtraction One-Step Equations
Addition and subtraction one-step equations involve adding or subtracting the same value from both sides of the equation to isolate the variable. For example, if we have the equation x + 3 = 7, we can solve for x by subtracting 3 from both sides, resulting in x = 4. Similarly, for the equation x - 2 = 5, adding 2 to both sides yields x = 7. These operations are based on the principle of inverse operations, where addition and subtraction are inverses of each other, as are multiplication and division.
| Type of Equation | Example | Solution |
|---|---|---|
| Addition | x + 4 = 9 | x = 9 - 4, x = 5 |
| Subtraction | x - 2 = 3 | x = 3 + 2, x = 5 |

Solving Multiplication and Division One-Step Equations
Multiplication and division one-step equations are solved by using the inverse operations of multiplication and division. For a multiplication equation like 3x = 12, dividing both sides by 3 gives x = 4. Conversely, for a division equation such as x / 2 = 6, multiplying both sides by 2 results in x = 12. These operations ensure that the variable is isolated on one side of the equation.
Practical Applications and Real-World Scenarios
One-step equations have numerous practical applications in real-world scenarios, making them a vital tool for problem-solving in various fields. In science, for instance, one-step equations can be used to calculate quantities such as speed, distance, or time when one of these variables is known. In finance, one-step equations are useful for calculating interest rates, investments, or the cost of goods after a discount. Understanding how to solve one-step equations empowers individuals to make informed decisions and solve problems efficiently in their daily lives.
Common Challenges and Tips
Despite their simplicity, one-step equations can sometimes pose challenges, especially for those new to algebra. A common mistake is forgetting to perform the inverse operation on both sides of the equation, which can lead to incorrect solutions. To overcome this, it’s essential to double-check work and ensure that the equation remains balanced throughout the solution process. Additionally, practicing a variety of one-step equations can help build confidence and fluency in solving these equations.
What is the primary goal when solving a one-step equation?
+The primary goal is to isolate the variable on one side of the equation, which is achieved by performing the appropriate inverse operation on both sides of the equation.
How do you solve a one-step equation involving multiplication?
+You solve it by dividing both sides of the equation by the coefficient of the variable. For example, in the equation 4x = 20, dividing both sides by 4 gives x = 5.
What is the importance of checking your work when solving one-step equations?
+Checking your work is crucial because it helps ensure that the solution is correct and that no errors were made during the solving process. This can be done by substituting the solution back into the original equation to verify that it holds true.
In conclusion, solving one-step equations is a foundational skill in mathematics that has wide-ranging applications in various fields. By understanding the principles of inverse operations and applying them correctly, individuals can efficiently solve one-step equations and build a strong foundation for more advanced mathematical concepts. Whether in academic, professional, or personal contexts, the ability to solve one-step equations is an invaluable tool for problem-solving and critical thinking.