Understanding the fundamentals of geometry is crucial for various applications in mathematics, physics, engineering, and other fields. Two essential concepts in geometry are parallel and perpendicular lines. Parallel lines are lines that lie in the same plane and never intersect, maintaining the same distance between them at all points. On the other hand, perpendicular lines are lines that intersect at a right angle, forming a 90-degree angle. In this article, we will delve into the properties, applications, and differences between parallel and perpendicular lines, exploring their significance in various contexts.
Key Points
- Parallel lines never intersect and maintain a constant distance between them.
- Perpendicular lines intersect at a right angle, forming a 90-degree angle.
- The properties of parallel and perpendicular lines are fundamental in geometry and trigonometry.
- Understanding parallel and perpendicular lines is crucial in various applications, including architecture, engineering, and design.
- The concept of parallel and perpendicular lines can be extended to higher dimensions, such as in 3D geometry and calculus.
Properties of Parallel Lines
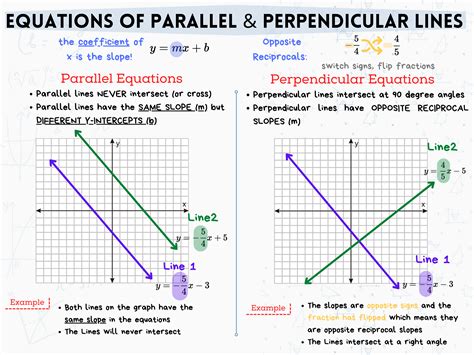
Parallel lines have several distinct properties that set them apart from other types of lines. One of the primary properties of parallel lines is that they never intersect, regardless of how far they are extended. This means that parallel lines will always maintain the same distance between them, never touching or crossing each other. Another key property of parallel lines is that they have the same slope, which is a measure of how steep a line is. In the context of coordinate geometry, parallel lines have the same slope, but different y-intercepts.
Applications of Parallel Lines
Parallel lines have numerous applications in various fields, including architecture, engineering, and design. In architecture, parallel lines are used to create symmetrical and balanced designs, such as in the construction of buildings and bridges. In engineering, parallel lines are used to design and analyze systems, such as in the development of electronic circuits and mechanical systems. In design, parallel lines are used to create visually appealing and balanced compositions, such as in graphic design and art.
| Property | Description |
|---|---|
| Slope | Parallel lines have the same slope. |
| Intersection | Parallel lines never intersect. |
| Distance | Parallel lines maintain a constant distance between them. |

Properties of Perpendicular Lines

Perpendicular lines, on the other hand, have distinct properties that set them apart from parallel lines. One of the primary properties of perpendicular lines is that they intersect at a right angle, forming a 90-degree angle. This means that perpendicular lines will always intersect, unless they are parallel. Another key property of perpendicular lines is that their slopes are negative reciprocals of each other, which means that the product of their slopes is -1.
Applications of Perpendicular Lines
Perpendicular lines have numerous applications in various fields, including physics, engineering, and design. In physics, perpendicular lines are used to describe the motion of objects, such as in the context of velocity and acceleration. In engineering, perpendicular lines are used to design and analyze systems, such as in the development of mechanical systems and electronic circuits. In design, perpendicular lines are used to create balanced and harmonious compositions, such as in graphic design and art.
| Property | Description |
|---|---|
| Intersection | Perpendicular lines intersect at a right angle. |
| Slope | Perpendicular lines have slopes that are negative reciprocals of each other. |
| Angle | Perpendicular lines form a 90-degree angle. |
What is the difference between parallel and perpendicular lines?
+Parallel lines never intersect and maintain a constant distance between them, while perpendicular lines intersect at a right angle, forming a 90-degree angle.
What are some applications of parallel and perpendicular lines?
+Parallel and perpendicular lines have numerous applications in various fields, including architecture, engineering, design, physics, and mathematics.
How are parallel and perpendicular lines used in geometry and trigonometry?
+Parallel and perpendicular lines are fundamental concepts in geometry and trigonometry, used to describe and analyze various geometric shapes and relationships.
In conclusion, parallel and perpendicular lines are two fundamental concepts in geometry, with distinct properties and applications. Understanding these concepts is crucial for various applications in mathematics, physics, engineering, and design. By recognizing the differences and similarities between parallel and perpendicular lines, individuals can develop a deeper appreciation for the underlying principles of geometry and their significance in various contexts.



