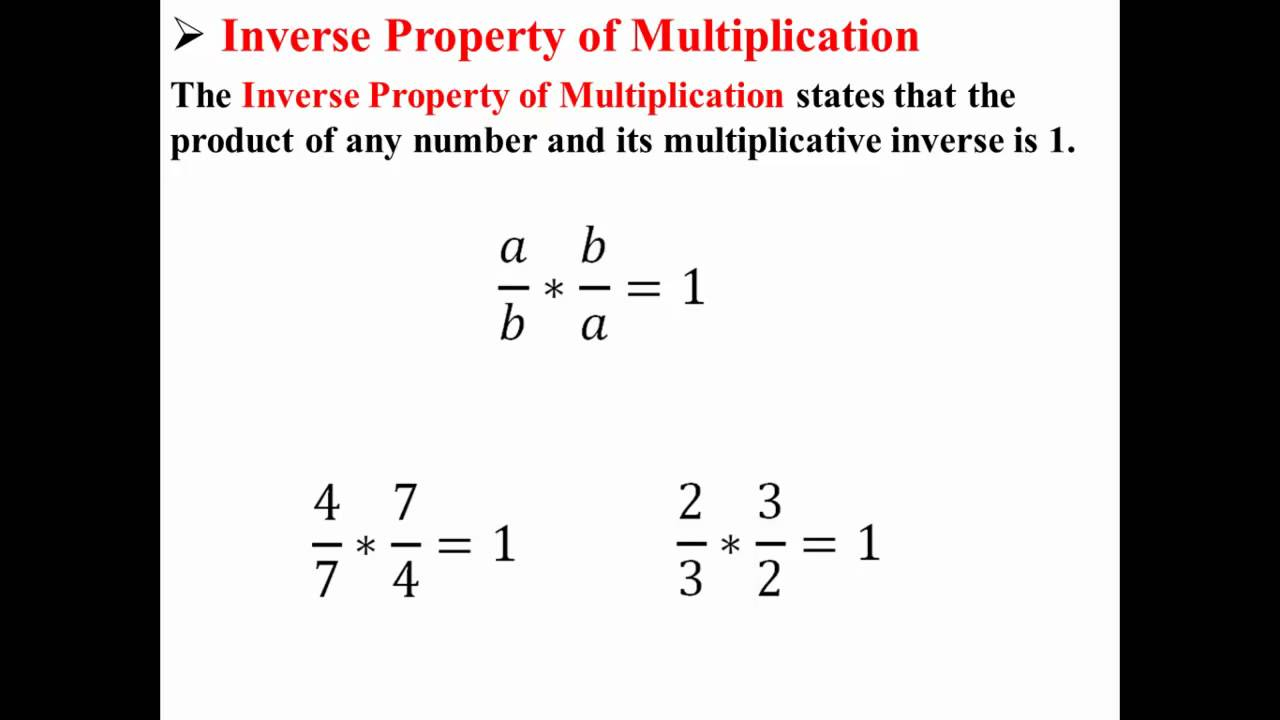
The multiplicative inverse property is a fundamental concept in mathematics, particularly in algebra and arithmetic. It states that for any non-zero number, there exists a multiplicative inverse, which when multiplied by the original number, results in the multiplicative identity, typically represented as 1. This property is crucial in various mathematical operations, including solving equations, simplifying expressions, and performing calculations involving fractions and decimals.
In essence, the multiplicative inverse of a number is its reciprocal, denoted as 1/x for a number x. For instance, the multiplicative inverse of 2 is 1/2, as 2 * (1/2) = 1. Similarly, the multiplicative inverse of -3 is -1/3, since -3 * (-1/3) = 1. This concept can be applied to all non-zero real numbers, including integers, fractions, and decimals.
Key Points
- The multiplicative inverse property states that every non-zero number has a multiplicative inverse.
- The multiplicative inverse of a number is its reciprocal, denoted as 1/x for a number x.
- The product of a number and its multiplicative inverse is always 1.
- This property is essential for solving equations, simplifying expressions, and performing calculations involving fractions and decimals.
- The multiplicative inverse property applies to all non-zero real numbers, including integers, fractions, and decimals.
Mathematical Representation and Examples

The multiplicative inverse property can be mathematically represented as x * (1/x) = 1, where x is a non-zero number. For example, if we have the number 4, its multiplicative inverse is 1⁄4, as 4 * (1⁄4) = 1. Similarly, the multiplicative inverse of -5 is -1⁄5, since -5 * (-1⁄5) = 1.
It's essential to note that the multiplicative inverse property does not apply to zero, as there is no number that can be multiplied by zero to result in 1. In fact, any number multiplied by zero results in zero, highlighting the unique properties of zero in arithmetic operations.
Application in Solving Equations
The multiplicative inverse property is particularly useful in solving linear equations, where it can be employed to isolate the variable. For instance, consider the equation 2x = 6. To solve for x, we can multiply both sides of the equation by the multiplicative inverse of 2, which is 1⁄2. This yields x = 6 * (1⁄2) = 3, demonstrating the application of the multiplicative inverse property in equation solving.
| Number | Multiplicative Inverse | Product |
|---|---|---|
| 2 | 1/2 | 2 * (1/2) = 1 |
| -3 | -1/3 | -3 * (-1/3) = 1 |
| 4 | 1/4 | 4 * (1/4) = 1 |
| -5 | -1/5 | -5 * (-1/5) = 1 |
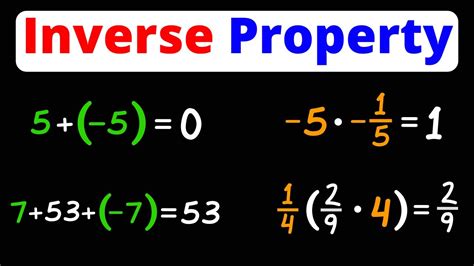
Real-World Applications and Implications

The multiplicative inverse property has numerous real-world applications, including science, engineering, and economics. For instance, in physics, the concept of multiplicative inverse is used to describe the relationship between force and distance, where the multiplicative inverse of force is used to calculate the distance traveled by an object. Similarly, in economics, the multiplicative inverse property is employed to analyze the relationship between price and demand, where the multiplicative inverse of price is used to calculate the demand for a particular good or service.
In conclusion, the multiplicative inverse property is a fundamental concept in mathematics, with far-reaching implications and applications in various fields. Its understanding and application are essential for solving equations, simplifying expressions, and performing calculations involving fractions and decimals. By recognizing the importance of this property, individuals can develop a deeper appreciation for the underlying structure of mathematics and its role in shaping our understanding of the world.
What is the multiplicative inverse property?
+The multiplicative inverse property states that every non-zero number has a multiplicative inverse, which when multiplied by the original number, results in the multiplicative identity, typically represented as 1.
How is the multiplicative inverse property used in solving equations?
+The multiplicative inverse property is used to isolate the variable in linear equations by multiplying both sides of the equation by the multiplicative inverse of the coefficient of the variable.
What are some real-world applications of the multiplicative inverse property?
+The multiplicative inverse property has numerous real-world applications, including science, engineering, and economics, where it is used to describe relationships between physical quantities, calculate distances, and analyze economic systems.
Meta Description: Learn about the multiplicative inverse property, its definition, examples, and real-world applications, and discover how it is used to solve equations and simplify expressions. (149 characters)