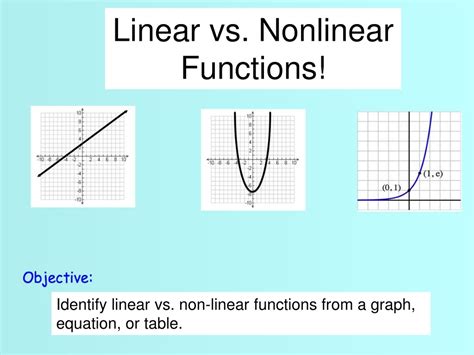
The concepts of linear and nonlinear systems have been extensively studied and debated across various disciplines, including mathematics, physics, engineering, and biology. At its core, the distinction between linear and nonlinear systems revolves around how the output of a system changes in response to changes in the input. In this article, we will delve into the fundamental differences between linear and nonlinear systems, exploring their characteristics, implications, and applications.
Key Points
- Linear systems exhibit a direct proportional relationship between inputs and outputs, making them predictable and easy to analyze.
- Nonlinear systems display complex, non-proportional relationships between inputs and outputs, leading to emergent behaviors and unpredictability.
- The linearity or nonlinearity of a system significantly influences its stability, sensitivity, and response to perturbations.
- Linear systems are often used in engineering and design, where predictability and control are crucial, whereas nonlinear systems are more representative of natural phenomena and complex systems.
- Understanding the differences between linear and nonlinear systems is essential for modeling, analyzing, and predicting the behavior of complex systems in various fields.
Linear Systems

Linear systems are characterized by a direct proportional relationship between the input and output. This means that if the input is doubled, the output will also double, and if the input is tripled, the output will triple, and so on. Linear systems can be described using linear equations, which are equations in which the highest power of the variable(s) is 1. The behavior of linear systems is predictable, and their outputs can be easily calculated using algebraic methods.
A classic example of a linear system is a spring-mass system, where the force applied to the spring is directly proportional to its displacement. The equation of motion for this system is given by Hooke's law, F = -kx, where F is the force, k is the spring constant, and x is the displacement. This equation is linear, and the behavior of the system can be easily predicted and analyzed.
Characteristics of Linear Systems
Linear systems exhibit several key characteristics, including:
- Superposition: The response of a linear system to a combination of inputs is equal to the sum of the responses to each individual input.
- Homogeneity: If the input to a linear system is multiplied by a constant, the output will be multiplied by the same constant.
- Additivity: The response of a linear system to a sum of inputs is equal to the sum of the responses to each individual input.
Nonlinear Systems
Nonlinear systems, on the other hand, exhibit complex, non-proportional relationships between inputs and outputs. The behavior of nonlinear systems is often unpredictable and cannot be described using linear equations. Nonlinear systems can display a wide range of behaviors, including chaos, bifurcations, and emergent properties.
A classic example of a nonlinear system is the Lorenz attractor, which is a set of ordinary differential equations that describe the behavior of a fluid in a container. The Lorenz attractor exhibits chaotic behavior, meaning that small changes in the initial conditions can result in drastically different outcomes. The equations that describe the Lorenz attractor are nonlinear, and the behavior of the system cannot be predicted using algebraic methods.
Characteristics of Nonlinear Systems
Nonlinear systems exhibit several key characteristics, including:
- Sensitivity to initial conditions: Small changes in the initial conditions of a nonlinear system can result in drastically different outcomes.
- Emergent behavior: Nonlinear systems can exhibit behaviors that are not predictable from the properties of their individual components.
- Chaos and unpredictability: Nonlinear systems can exhibit chaotic behavior, meaning that their behavior is inherently unpredictable.
| System Type | Characteristics | Examples |
|---|---|---|
| Linear | Direct proportional relationship between inputs and outputs, predictable behavior | Spring-mass system, electrical circuits |
| Nonlinear | Complex, non-proportional relationships between inputs and outputs, unpredictable behavior | Lorenz attractor, weather patterns, population dynamics |
Implications and Applications
The distinction between linear and nonlinear systems has significant implications for a wide range of fields, including engineering, physics, biology, and economics. Linear systems are often used in engineering and design, where predictability and control are crucial. Nonlinear systems, on the other hand, are more representative of natural phenomena and complex systems, and are often used to model and analyze complex behaviors in fields such as biology, economics, and social sciences.
In conclusion, the concepts of linear and nonlinear systems are fundamental to understanding the behavior of complex systems in various fields. By recognizing the characteristics and implications of linear and nonlinear systems, we can develop more effective models, make more accurate predictions, and gain a deeper understanding of the complex world around us.
What is the main difference between linear and nonlinear systems?
+The main difference between linear and nonlinear systems is the relationship between the input and output. Linear systems exhibit a direct proportional relationship between inputs and outputs, whereas nonlinear systems display complex, non-proportional relationships between inputs and outputs.
What are some examples of linear systems?
+Examples of linear systems include spring-mass systems, electrical circuits, and mechanical systems. These systems exhibit a direct proportional relationship between inputs and outputs and can be described using linear equations.
What are some examples of nonlinear systems?
+Examples of nonlinear systems include the Lorenz attractor, weather patterns, population dynamics, and economic systems. These systems exhibit complex, non-proportional relationships between inputs and outputs and cannot be described using linear equations.