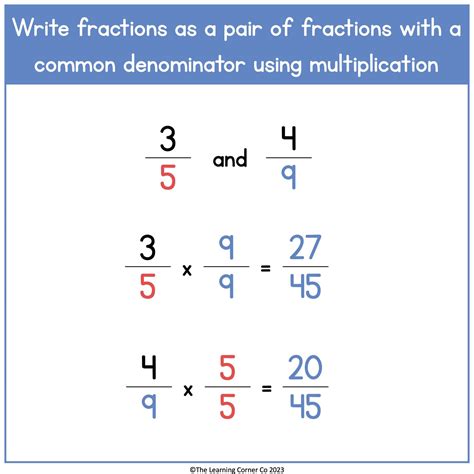
When working with fractions, finding a common denominator is a crucial step in adding, subtracting, multiplying, or dividing them. The concept of a common denominator may seem daunting at first, but it's actually a straightforward process that can be mastered with practice. In this article, we'll delve into the world of fractions, exploring the concept of common denominators, and provide a step-by-step guide on how to find them.
Understanding Fractions and Denominators

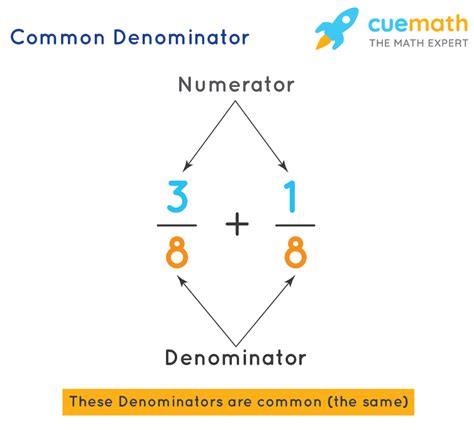
A fraction is a way of expressing a part of a whole as a ratio of two numbers. The top number, known as the numerator, represents the number of equal parts, while the bottom number, known as the denominator, represents the total number of parts. For example, in the fraction 3⁄4, the numerator is 3, and the denominator is 4. To find a common denominator, we need to identify the least common multiple (LCM) of the denominators involved.
What is the Least Common Multiple (LCM)?
The LCM is the smallest number that is a multiple of two or more numbers. To find the LCM of two numbers, we can list the multiples of each number and identify the smallest multiple they have in common. For instance, the multiples of 4 are 4, 8, 12, 16, and so on, while the multiples of 6 are 6, 12, 18, 24, and so on. The smallest multiple that appears in both lists is 12, making it the LCM of 4 and 6.
| Denominator | Multiples |
|---|---|
| 4 | 4, 8, 12, 16,... |
| 6 | 6, 12, 18, 24,... |
Step-by-Step Guide to Finding a Common Denominator
Now that we’ve covered the basics of fractions and LCMs, let’s move on to the step-by-step guide on finding a common denominator.
Step 1: Identify the Denominators
The first step is to identify the denominators of the fractions involved. For example, if we have the fractions 1⁄4 and 1⁄6, the denominators are 4 and 6, respectively.
Step 2: Find the LCM of the Denominators
Next, we need to find the LCM of the denominators. Using the example above, we would list the multiples of 4 and 6 to find their LCM, which is 12.
Step 3: Convert Each Fraction to Have the Common Denominator
Once we have the LCM, we can convert each fraction to have the common denominator. To do this, we multiply the numerator and denominator of each fraction by the necessary multiple. For instance, to convert 1⁄4 to have a denominator of 12, we would multiply both the numerator and denominator by 3, resulting in 3⁄12.
| Fraction | Common Denominator | Equivalent Fraction |
|---|---|---|
| 1/4 | 12 | 3/12 |
| 1/6 | 12 | 2/12 |
Key Points
- The common denominator is the least common multiple (LCM) of the denominators involved.
- To find the LCM, we can list the multiples of each number or use the prime factorization method.
- Once we have the LCM, we can convert each fraction to have the common denominator by multiplying the numerator and denominator by the necessary multiple.
- Converting fractions to have a common denominator allows us to add, subtract, multiply, or divide them easily.
- Practice makes perfect, so be sure to practice finding common denominators with different fractions and denominators.
Practical Applications and Examples
Finding common denominators has numerous practical applications in real-life scenarios. For instance, when measuring ingredients for a recipe, we may need to add or subtract fractions with different denominators. By finding a common denominator, we can easily perform these operations and ensure accurate measurements.
Example 1: Adding Fractions with Different Denominators
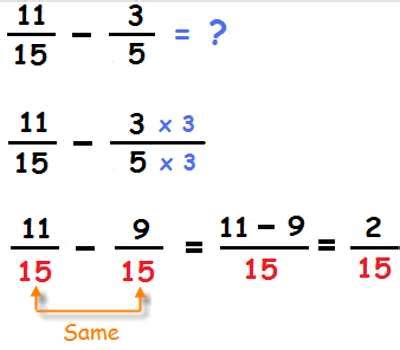
Suppose we want to add 1⁄4 and 1⁄6. To do this, we would first find the LCM of 4 and 6, which is 12. Then, we would convert each fraction to have a denominator of 12, resulting in 3⁄12 and 2⁄12. Finally, we can add the fractions: 3⁄12 + 2⁄12 = 5⁄12.
Example 2: Subtracting Fractions with Different Denominators
Let’s say we want to subtract 1⁄6 from 1⁄4. Again, we would find the LCM of 4 and 6, which is 12. Then, we would convert each fraction to have a denominator of 12, resulting in 3⁄12 and 2⁄12. Finally, we can subtract the fractions: 3⁄12 - 2⁄12 = 1⁄12.
What is the purpose of finding a common denominator?
+The purpose of finding a common denominator is to allow us to add, subtract, multiply, or divide fractions easily. By converting fractions to have the same denominator, we can perform these operations without having to worry about different denominators.
How do I find the LCM of two numbers?
+To find the LCM of two numbers, we can list the multiples of each number and identify the smallest multiple they have in common. Alternatively, we can use the prime factorization method, which involves breaking down each number into its prime factors and taking the highest power of each prime factor that appears in any of the numbers.
What is the difference between the LCM and the greatest common divisor (GCD)?
+The LCM is the smallest number that is a multiple of two or more numbers, while the GCD is the largest number that divides two or more numbers without leaving a remainder. In other words, the LCM is the "least common multiple," while the GCD is the "greatest common divisor."
In conclusion, finding common denominators is an essential skill for working with fractions. By understanding the concept of LCMs and following the step-by-step guide, we can easily find common denominators and perform various operations with fractions. Remember to practice, practice, practice, and you’ll become a pro at finding common denominators in no time!