The differentiation of trigonometric functions is a fundamental concept in calculus, allowing us to find the rates of change of these functions with respect to their input variables. Trigonometric functions, such as sine, cosine, and tangent, are used to describe the relationships between the angles and side lengths of triangles, and their derivatives are crucial in various fields, including physics, engineering, and navigation. In this article, we will delve into the differentiation of trig functions, exploring the rules, formulas, and applications of these derivatives.
Key Points
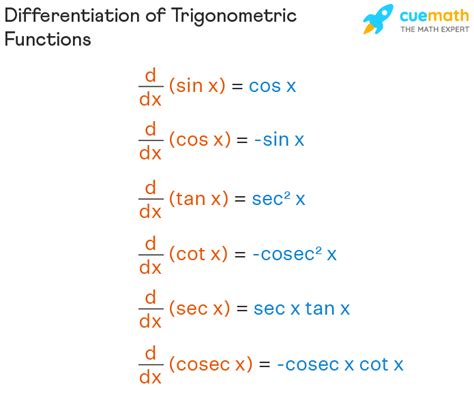
- The derivative of the sine function is the cosine function, with a derivative of cos(x).
- The derivative of the cosine function is the negative sine function, with a derivative of -sin(x).
- The derivative of the tangent function is the secant squared function, with a derivative of sec^2(x).
- Trigonometric derivatives have numerous applications in physics, engineering, and navigation, including the description of simple harmonic motion and the calculation of wave velocities.
- The chain rule and product rule are essential for differentiating composite trigonometric functions and functions involving trigonometric expressions.
Derivatives of Basic Trigonometric Functions

The derivatives of the basic trigonometric functions are as follows:
The derivative of sin(x) is cos(x).
The derivative of cos(x) is -sin(x).
The derivative of tan(x) is sec^2(x).
These derivatives can be proven using the definition of a derivative and the trigonometric identities. For example, the derivative of sin(x) can be proven using the limit definition of a derivative and the angle sum formula for sine.
Derivative of Sine
The derivative of sin(x) is cos(x). This can be proven using the following limit:
lim(h → 0) [sin(x + h) - sin(x)]/h = lim(h → 0) [sin(x)cos(h) + cos(x)sin(h) - sin(x)]/h
= lim(h → 0) [sin(x)(cos(h) - 1) + cos(x)sin(h)]/h
= cos(x)
This result can be used to find the derivatives of more complex functions involving sine, such as sin(2x) or sin(x^2).
Derivative of Cosine
The derivative of cos(x) is -sin(x). This can be proven using a similar limit:
lim(h → 0) [cos(x + h) - cos(x)]/h = lim(h → 0) [cos(x)cos(h) - sin(x)sin(h) - cos(x)]/h
= lim(h → 0) [cos(x)(cos(h) - 1) - sin(x)sin(h)]/h
= -sin(x)
This result can be used to find the derivatives of more complex functions involving cosine, such as cos(2x) or cos(x^2).
Derivative of Tangent
The derivative of tan(x) is sec^2(x). This can be proven using the quotient rule and the derivatives of sine and cosine:
d(tan(x))/dx = d(sin(x)/cos(x))/dx
= (cos(x)(cos(x)) - sin(x)(-sin(x)))/cos^2(x)
= (cos^2(x) + sin^2(x))/cos^2(x)
= 1/cos^2(x) = sec^2(x)
This result can be used to find the derivatives of more complex functions involving tangent, such as tan(2x) or tan(x^2).
| Trigonometric Function | Derivative |
|---|---|
| sin(x) | cos(x) |
| cos(x) | -sin(x) |
| tan(x) | sec^2(x) |
| sec(x) | sec(x)tan(x) |
| csc(x) | -csc(x)cot(x) |
| cot(x) | -csc^2(x) |

Applications of Trigonometric Derivatives

Trigonometric derivatives have numerous applications in physics, engineering, and navigation. Some examples include:
Simple Harmonic Motion: The derivative of the sine function is used to describe the velocity of a pendulum, while the derivative of the cosine function is used to describe the velocity of a spring-mass system.
Wave Motion: The derivatives of trigonometric functions are used to describe the motion of waves, including water waves, sound waves, and light waves.
Navigation: Trigonometric derivatives are used in navigation to calculate the position and velocity of objects, including ships, aircraft, and spacecraft.
Derivatives of Composite Trigonometric Functions
The derivatives of composite trigonometric functions, such as sin(2x) or cos(x^2), can be found using the chain rule and the product rule. For example:
d(sin(2x))/dx = 2cos(2x)
d(cos(x^2))/dx = -2xsin(x^2)
These results can be used to find the derivatives of more complex functions involving trigonometric expressions.
What is the derivative of the sine function?
+The derivative of the sine function is the cosine function, with a derivative of cos(x).
What is the derivative of the cosine function?
+The derivative of the cosine function is the negative sine function, with a derivative of -sin(x).
What is the derivative of the tangent function?
+The derivative of the tangent function is the secant squared function, with a derivative of sec^2(x).
In conclusion, the differentiation of trigonometric functions is a fundamental concept in calculus, with numerous applications in physics, engineering, and navigation. The derivatives of the basic trigonometric functions, including sine, cosine, and tangent, can be proven using the definition of a derivative and the trigonometric identities. These derivatives can be used to find the derivatives of more complex functions involving trigonometric expressions, and have numerous applications in the description of simple harmonic motion, wave motion, and navigation.