The concept of De Broglie wavelength, proposed by Louis de Broglie in 1924, revolutionized the field of quantum mechanics by suggesting that particles, such as electrons, exhibit wave-like behavior. This idea was a pivotal moment in the development of modern physics, as it challenged the long-held notion that particles and waves were distinct entities. De Broglie's hypothesis, which posits that any particle with momentum (p) has a wavelength (λ) associated with it, can be expressed through the equation λ = h / p, where h is Planck's constant.
Historical Context and Development
The development of the De Broglie wavelength concept was influenced by earlier work in physics, particularly the discovery of wave-particle duality. Experiments such as the double-slit experiment, which demonstrated the wave-like behavior of light, laid the groundwork for de Broglie’s proposal. By extending this principle to particles with mass, de Broglie provided a fundamental explanation for the observed behaviors in atomic and subatomic physics, such as the quantization of energy levels in atoms. This concept was later experimentally confirmed through electron diffraction experiments, solidifying its place in quantum theory.
Mathematical Formulation and Implications
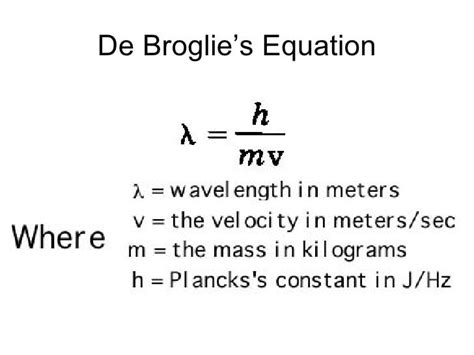
The mathematical formulation of the De Broglie wavelength, λ = h / p, introduces a direct relationship between the particle’s momentum and its associated wavelength. For particles such as electrons, which have a small mass and can exhibit significant wave-like properties due to their low momentum, the De Broglie wavelength can be substantial. This has profound implications for understanding phenomena at the atomic and subatomic level, including the structure of atoms and the behavior of particles in potential wells. The equation also highlights the inverse relationship between wavelength and momentum, suggesting that as the momentum of a particle increases, its wavelength decreases, making wave-like behavior less observable.
| Particle | Momentum (kg·m/s) | De Broglie Wavelength (m) |
|---|---|---|
| Electron | 1.0 × 10^-24 | 7.6 × 10^-10 |
| Proton | 1.0 × 10^-20 | 7.6 × 10^-13 |

Applications and Implications in Modern Physics
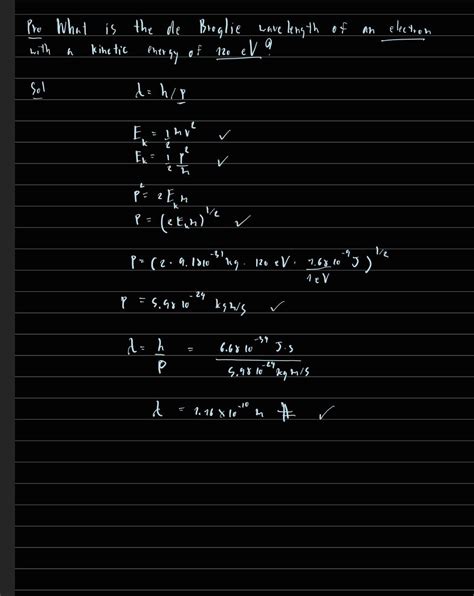
The concept of De Broglie wavelength has numerous applications in modern physics, including the design of electronic devices, the study of materials at the nanoscale, and the development of quantum computing. In electron microscopy, for instance, the De Broglie wavelength of electrons is exploited to achieve high-resolution imaging, surpassing the limitations of traditional light microscopy. Furthermore, the understanding of wave-particle duality has been crucial in the advancement of semiconductor technology, where the manipulation of electron waves is essential for the operation of devices such as transistors and diodes.
Critical Analysis and Future Directions
A critical analysis of the De Broglie wavelength concept reveals its significance in bridging the gap between classical mechanics and quantum mechanics. However, it also poses challenges in understanding the nature of reality at the quantum level, particularly in reconciling wave-like behavior with particle-like properties. Future research directions may involve further exploration of quantum systems, the development of new materials with tailored quantum properties, and the pursuit of quantum computing technologies that can harness and manipulate wave-like behavior for computational purposes.
Key Points
- The De Broglie wavelength relates the momentum of a particle to its wave-like behavior, expressed as λ = h / p.
- This concept is fundamental to understanding quantum mechanics and has been experimentally verified through electron diffraction experiments.
- Applications of the De Broglie wavelength include electron microscopy, semiconductor technology, and the development of quantum computing.
- The concept poses challenges in understanding the nature of reality at the quantum level and requires further research into quantum systems and materials.
- The De Broglie hypothesis has led to significant technological innovations and continues to be a cornerstone of modern physics.
In conclusion, the De Broglie wavelength represents a landmark concept in the history of physics, offering a profound insight into the nature of particles and waves. Its implications and applications continue to shape our understanding of the quantum world and drive technological innovation. As research into quantum mechanics and its applications progresses, the De Broglie wavelength remains a foundational principle, inspiring new discoveries and deeper understanding of the intricate relationships between particles, waves, and the fabric of reality.
What is the significance of the De Broglie wavelength in modern physics?
+The De Broglie wavelength is significant because it explains the wave-like behavior of particles, which is a fundamental aspect of quantum mechanics. It has led to numerous technological innovations, including the development of transistors, electron microscopes, and has potential applications in quantum computing.
How does the De Broglie wavelength relate to the double-slit experiment?
+The double-slit experiment demonstrates the wave-like behavior of particles, such as electrons. The De Broglie wavelength provides a mathematical formulation for this behavior, relating the particle's momentum to its wavelength. This concept is essential for understanding the interference patterns observed in the double-slit experiment.
What are the potential future directions for research involving the De Broglie wavelength?
+Future research directions may include the exploration of quantum systems, the development of new materials with tailored quantum properties, and the advancement of quantum computing technologies. Understanding and manipulating the De Broglie wavelength of particles will be crucial in these endeavors.
Meta Description: Discover the significance of the De Broglie wavelength in quantum mechanics, its historical development, and its applications in modern physics, from electron microscopy to quantum computing.