Algebra, a fundamental branch of mathematics, deals with the study of variables and their relationships. It is a critical subject for students, as it lays the groundwork for advanced mathematical concepts and problem-solving skills. Mastering algebra can seem daunting, but with the right approach and strategies, it can become more accessible and even enjoyable. In this article, we will delve into five essential algebra tips to help students improve their understanding and performance in this subject.
Key Points
- Understand the basics of algebra, including variables, constants, and mathematical operations
- Practice solving linear equations and inequalities to develop problem-solving skills
- Learn to graph linear equations and functions to visualize relationships between variables
- Apply algebraic concepts to real-world problems to appreciate their practical significance
- Use online resources and study groups to supplement classroom instruction and stay motivated
Tip 1: Master the Basics of Algebra

Before diving into complex algebraic concepts, it is crucial to have a solid grasp of the basics. This includes understanding variables, constants, and mathematical operations such as addition, subtraction, multiplication, and division. Students should be able to simplify expressions, solve basic equations, and work with fractions and decimals. Practice is key in reinforcing these fundamental skills, and students can use online resources or worksheets to reinforce their understanding.
Understanding Variables and Constants
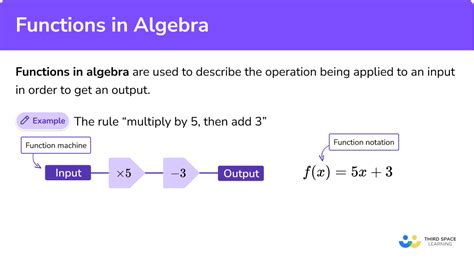
In algebra, variables are letters or symbols that represent unknown values or quantities that can change. Constants, on the other hand, are numbers or values that remain fixed. Understanding the difference between variables and constants is vital, as it forms the foundation of algebraic expressions and equations. For example, in the equation 2x + 5 = 11, x is a variable, and 5 and 11 are constants.
Tip 2: Practice Solving Linear Equations and Inequalities

Linear equations and inequalities are a crucial part of algebra, and practicing their solutions can help students develop problem-solving skills. Linear equations are equations in which the highest power of the variable is 1, and they can be written in the form ax + b = c, where a, b, and c are constants. Inequalities, on the other hand, are statements that compare two expressions using greater than, less than, greater than or equal to, or less than or equal to. Students should practice solving these equations and inequalities to develop their critical thinking and analytical skills.
| Type of Equation | Example |
|---|---|
| Linear Equation | 2x + 3 = 7 |
| Linear Inequality | 2x - 5 > 3 |

Tip 3: Learn to Graph Linear Equations and Functions
Graphing linear equations and functions is an essential skill in algebra, as it helps students visualize the relationships between variables. Graphs can be used to identify the x-intercept, y-intercept, and slope of a line, as well as to determine the equation of a line. Students can use online graphing tools or graphing calculators to practice graphing linear equations and functions. For instance, the equation y = 2x + 3 can be graphed using a coordinate plane, and students can use this graph to identify the x-intercept, y-intercept, and slope of the line.
Tip 4: Apply Algebraic Concepts to Real-World Problems
Algebra is not just a theoretical subject; it has numerous practical applications in real-world problems. Students can apply algebraic concepts to problems in physics, engineering, economics, and computer science, among other fields. Real-world problems can help students appreciate the significance and relevance of algebra, making it more engaging and motivating. For example, algebra can be used to model population growth, optimize business processes, and analyze financial data.
Tip 5: Use Online Resources and Study Groups to Supplement Classroom Instruction
Finally, students can use online resources and study groups to supplement classroom instruction and stay motivated. Online resources such as Khan Academy, MIT OpenCourseWare, and Wolfram Alpha can provide students with additional practice problems, video lectures, and interactive simulations. Study groups can also provide students with a supportive community of peers who can collaborate on homework assignments, discuss challenging concepts, and share study tips and strategies.
What are some common mistakes to avoid when solving algebraic equations?
+Common mistakes to avoid when solving algebraic equations include failing to distribute coefficients, neglecting to combine like terms, and forgetting to check the solution. Students should also be careful when working with negative numbers and fractions.
How can I use algebra to solve real-world problems?
+Algebra can be used to solve real-world problems by modeling relationships between variables, optimizing processes, and analyzing data. Students can apply algebraic concepts to problems in physics, engineering, economics, and computer science, among other fields.
What are some online resources available for learning algebra?
+Online resources available for learning algebra include Khan Academy, MIT OpenCourseWare, and Wolfram Alpha. These resources provide students with additional practice problems, video lectures, and interactive simulations to supplement classroom instruction.
In conclusion, mastering algebra requires a combination of understanding the basics, practicing problem-solving skills, and applying algebraic concepts to real-world problems. By following these five algebra tips, students can develop a deeper understanding of algebra and improve their performance in this subject. Remember to practice regularly, use online resources and study groups, and apply algebraic concepts to real-world problems to appreciate their practical significance. With persistence and dedication, students can overcome the challenges of algebra and develop a strong foundation for advanced mathematical concepts and problem-solving skills.
