The 45 45 90 triangle is a special right triangle with angles measuring 45, 45, and 90 degrees. This triangle is also known as an isosceles right triangle, given that two of its sides are equal in length. The 45 45 90 triangle rules are essential in various mathematical and real-world applications, including geometry, trigonometry, and engineering. Understanding these rules enables individuals to solve problems related to these triangles efficiently.
Key Points
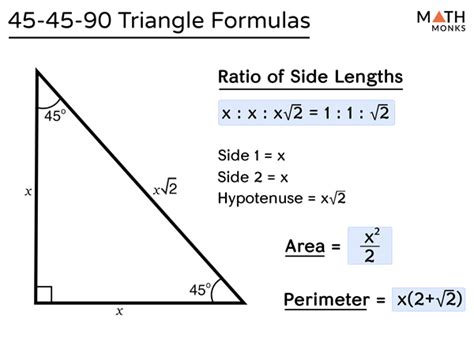
- The 45 45 90 triangle has two equal sides, known as legs, and a hypotenuse that is √2 times the length of a leg.
- The ratio of the sides in a 45 45 90 triangle is 1:1:√2, where the first two values represent the lengths of the legs and the third value represents the length of the hypotenuse.
- The area of a 45 45 90 triangle can be calculated using the formula A = (1/2) * base * height, where the base and height are equal in length.
- The 45 45 90 triangle is commonly used in design and construction, particularly in the creation of symmetrical structures and patterns.
- Trigonometric ratios, such as sine, cosine, and tangent, can be easily calculated for 45 45 90 triangles using the ratio of the sides.
Understanding the 45 45 90 Triangle
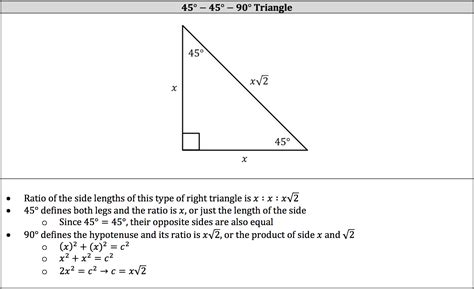
A 45 45 90 triangle is a right triangle with two equal acute angles, each measuring 45 degrees. The side opposite the 90-degree angle is called the hypotenuse, and the other two sides are called legs. Since the triangle is isosceles, the two legs are equal in length. The hypotenuse, which is the longest side, is equal to the length of a leg multiplied by √2.
Ratio of Sides in a 45 45 90 Triangle
The ratio of the sides in a 45 45 90 triangle is 1:1:√2. This means that if the length of a leg is x, then the length of the hypotenuse is x√2. This ratio is essential in solving problems related to these triangles, as it allows for the calculation of unknown side lengths. For example, if the length of a leg is 5 units, then the length of the hypotenuse is 5√2 units.
| Side | Length |
|---|---|
| Leg | x |
| Leg | x |
| Hypotenuse | x√2 |
Applications of the 45 45 90 Triangle
The 45 45 90 triangle has numerous applications in mathematics, science, and engineering. Its properties make it an ideal shape for designing symmetrical structures and patterns. In construction, the 45 45 90 triangle is used to create strong and stable frameworks. In electronics, the triangle is used to design circuits and components. The 45 45 90 triangle is also used in trigonometry to calculate trigonometric ratios, such as sine, cosine, and tangent.
Trigonometric Ratios in a 45 45 90 Triangle
Trigonometric ratios can be easily calculated for 45 45 90 triangles using the ratio of the sides. The sine, cosine, and tangent of 45 degrees are all equal to 1/√2 or √2/2. This property makes it simple to calculate trigonometric ratios for these triangles. For example, if the length of a leg is x, then the sine of 45 degrees is x/x√2 = 1/√2.
In conclusion, the 45 45 90 triangle is a fundamental concept in mathematics, and its applications extend beyond basic geometry. Understanding the properties and rules of this triangle is essential to solve complex problems in various fields. The 45 45 90 triangle rules, including the ratio of sides and trigonometric ratios, are crucial in designing symmetrical structures and patterns, as well as in calculating unknown side lengths and trigonometric ratios.
What is the ratio of the sides in a 45 45 90 triangle?
+The ratio of the sides in a 45 45 90 triangle is 1:1:√2, where the first two values represent the lengths of the legs and the third value represents the length of the hypotenuse.
How do you calculate the area of a 45 45 90 triangle?
+The area of a 45 45 90 triangle can be calculated using the formula A = (1⁄2) * base * height, where the base and height are equal in length.
What are some common applications of the 45 45 90 triangle?
+The 45 45 90 triangle has numerous applications in mathematics, science, and engineering, including designing symmetrical structures and patterns, calculating trigonometric ratios, and creating strong and stable frameworks.
